Experimental Mathematics Lab at the University of Luxembourg

Congruence theorems for convex polygons involving medians and bisectors (show description)

Goal:

In this project we will explore the congruence of convex polygons in the Euclidean plane. The goal is to find geometric data that suffices to determine the polygon. This is a very classical type of problem in geometry, often referred to as a moduli problem.

Let's be a bit more precise. A polygon is a subset of the plane bounded by Euclidean line segments, and it is convex whenever the segment connecting any two points in the polygon is contained in the polygon. We say two polygons are congruent if all of the side lengths and angles are the same. A convex $n$-gon is a convex polygon of n sides. We will distinguish convex $n$-gons up to congruence, that is, consider two convex $n$-gons to be the same if and only if they are congruent to each other. This breaks up the set of convex $n$-gons, into subsets called congruence classes. The goal of this project is to find data that completely determines the congruence class of a convex $n$-gon.

One important piece of data is the set of medians, by which we mean the segments joining a vertex to the midpoint of a side (excluding the two neighboring sides). We also consider the bisectors, meaning the intersection with the polygon of the bisectors of the inner angles.

The fundamental question we will be studying is: if we only know the lenghts of the medians, is our convex $n$-gon determined up to congruence? Same question with the bisectors. We can also vary the assumptions a bit, which allows to produce easier statements to be proven.

We will clearly start with triangles (we have shown that the knowing the three medians determines the triangle up to congruence), and then move on to convex quadrilaterals. Studying such lower-complexity polygons should illustrate the basic definitions and help us see how the complexity of the situation bumps up once we increase the number of sides.

supervisors: Antonella Perucca, Didac Martinez Granado

Formalizing mathematics in Lean (show description)

Goal:

In recent years the idea of formal proof verification has really taken off. It consists of explaining to a computer how a proof goes, and have it check that every step is indeed fully justified. In this project you will learn the basics of Lean, a very user-friendly proof assistant, in which we will formalise proofs of some of the results you have seen in your courses.

Some experience with programming languages will be helpful.

By playing the Natural Numbers Game you can get an idea of what to expect in this project.

supervisors: Thilo Baumann, Pieter Belmans

Conway's game of life (show description)

Goal:

Conway's game of life is a famous cellular automaton, which leads to lots of interesting phenomena.

The goal of this project is to implement the Game of Life in SageMath, and create both two-dimensional and three-dimensional visualizations. The latter can show how a game has evolved over time, and we will use this visualization method to illustrate various interesting phenomena in the Game of Life, such as oscillators.

Some experience with programming languages will be helpful.

supervisor: Pieter Belmans

Drum resonance (show description)

Goal:

A drum's resonance is modeled by a wave equation on a circular membrame with fixed boundary. In playing, it can be varied by the change of the striking position, and by applying pressure to the drum-head. Particularly interesting choices are when the skin is pressed in the nodes (fixed points) of a chosen vibration mode, and when the drum is hit on its atinode (maximally vibrating point). The result seems to be that the choosen mode becomes dominant in the overall drum's sound.

Student(s) will do a computer simulation of the vibrating membrane subject to the above conditions, and compare to the experiment (audio recording).

Literature:

W. Strauss, Partial differential equations: An introduction, John Wiley & Sons, Inc., 1992
T. D. Rossing, Science of Percussion Instruments, Series in popular science – Volume 3, World Scientific Publishing, 2020

supervisor: Damjan Pistalo

The zeros of random functions: attraction, repulsion and other related properties (show description)

Goal:

The goal of this project is to study the behavior of the zeros set of a random function following a given distribution and comment about the 'shape': do the zeros tend to cluster or to repulse, compared to a Poisson point process. Many models can be studied : zeros of random polynomials with Gaussian coefficients, eigenvalues of random matrices, critical points of random fields, and even optimal transport between roots of a random polynomial and root of its derivative. The goal is to approach some theoretical results from a computational point of view and get (very) nice visual pictures. The literature around this topic is large and the direction of the project will be discussed between the teacher and the student(s).

We will need some basic probabilities and a bit of Gaussian analysis. A bit of computational skills will be useful, especially for plotting pictures.

supervisor: Louis Gass

Billiards in a cube and tetrahedral packings (description)

supervisor: Alexey Balitskiy

Laplacians on surfaces (show description)

Goal:

The Laplacian on a surface is a linear map that encodes essential information about the geometry of the surface and the behavior of various physical phenomena like heat flow. Among many applications of the Laplacian, we focus on the use of eigenvalues to detect the shape of objects.

Students will compute the eigenvalues of the Laplacian on various surfaces numerically. Along with the computational experiments, students will also learn the theory of the Laplacian.

Literature: Martin Reuter, Franz-Erich Wolter, Niklas Peinecke. Laplace–Beltrami spectra as ‘Shape-DNA’ of surfaces and solids. Computer-Aided Design, Volume 38, Issue 4, 2006, Pages 342-366

supervisor: Wai Yeung Lam

Distribution of roots of integer polynomials (show description)

Goal:

The picture

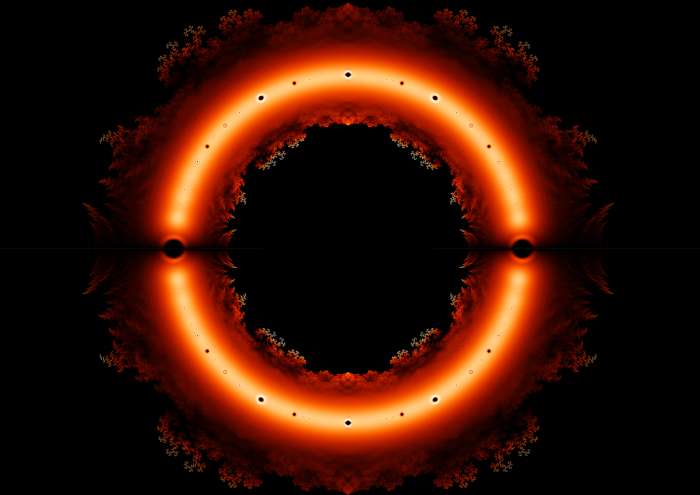
shows the zeros of the polynomials of degree at most 24 with coefficients either 1 or -1. A discussion around this fascinating topic of the (visualisation of the) distribution of roots of integral polynomials is available.

In this edition of the project, we would like to focus more on new interesting features:

Precisely determine zero-free regions, especially the 'holes' on the unit circle.
Understand the difference between real and complex roots.
Vary the sets of polynomials.

The project will be a computer experimentation with integral polynomials and their roots, accompanied by some theoretical considerations (e.g. via Taylor expansions centred at the 'holes'). Students might start by reproducing (some simple version of) some pictures found on the mentioned websites, and then create their own ones as variations.

supervisor: Gabor Wiese

Magic bodies (show description)

Goal:

Magic squares are famous objects of recreational mathematics: square matrices with (distinct positive) integer entries such that the sum of the numbers in each row, in each column and in the two main diagonals always equals the same number. One can simply obtain magic squares by solving systems of linear equations (but over the integers).

The goal of this project is to define and find 3-dimensional magic objects such as magic balls, magic tori, etc. via triangulations or other tilings. Furthermore, tangible 3d objects shall be created.

The mathematics will mostly be linear algebra (over the integers), but it will likely also involve some basic topology because the Euler characteristic might give some constraints (depending on your definition of being magic).

supervisor: Gabor Wiese